M408M Learning Module Pages
Main page

Position, velocity and acceleration

Position, Velocity, and Acceleration We now apply the machinery of vector-valued functions to understand motion in space, and the geometry of space curves. In fact, much of the application of mathematics to science can be traced back to Kepler's Laws describing the motion of the planets in terms of conic sections. Shortly afterwards Newton took these geometric descriptions and then created calculus to express the results analytically! Newton's Laws of Motion and the Theory of Gravitation followed. Much of vector calculus was developed in the $19^{\hbox{th}}$-century to explain newly emerging fields of electricity and magnetism, and Einstein later used calculus over surfaces to develop his theory of Relativity.

Recall that when $${\bf r}(t) \ = \ x(t)\,{\bf i} + y(t)\,{\bf j} + z(t)\,{\bf k}$$ is a vector function whose values are vectors in ${\mathbb R}^3$, then a space curve is simply the path traced out by the tip of ${\bf r}(t)$ as $t$ varies. If this path is thought of as the trajectory of an object moving in space, the parameter $t$ is naturally time, so that the tip of ${\bf r}(t)$ gives the position of the object at time $t$. In terms of motion in space, the examples that we saw earlier,

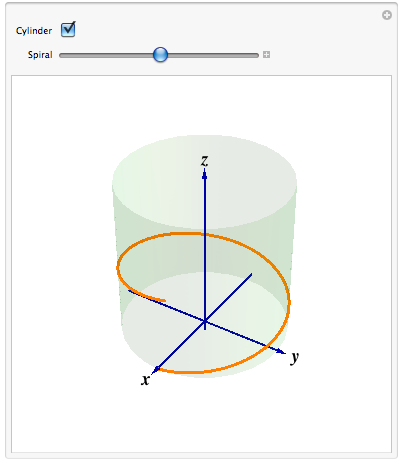${\bf r}(t) = \langle \cos t,\, \sin t, t \rangle$ ${\bf r}(t) = \langle t \cos t,\, t \sin t, t \rangle$

might be motion on a spiral staircase or the path of an object caught up in a tornado so that it spirals around a cone.

Again as we saw earlier, differentiation of a vector function is done one variable at a time: the first order and second order derivatives of ${\bf r}(t)$ are $${\bf r}' (t)\ = \ x'(t)\, {\bf i} + y'(t)\, {\bf j} + z'(t)\, {\bf k}\,, \qquad {\bf r}''(t)\ = \ x''(t)\, {\bf i} + y''(t)\, {\bf j} + z''(t)\, {\bf k}\,.$$ Both are vector functions; the first derivative ${\bf v}(t)={\bf r}'(t)$ is the velocity of the moving object, while the second derivative ${\bf a}(t)={\bf r}''(t)$ is its acceleration. Newton, of course, was really interested in starting with the acceleration!

Example 1: Find the position ${\bf r}(t)$ of a particle with acceleration $${\bf a}(t) \,=\, 2\, {\bf i} + 12 t\, {\bf j}\,,$$ when its initial velocity and position satisfy $${\bf v} (0)\,=\, 7\, {\bf i}, \quad {\bf r}(0)\,=\, 2\, {\bf i} + 9 {\bf k}\,.$$ Solution: Since ${\bf a}(t) = {\bf v}'(t)$, integration gives $${\bf v}(t)\, = {\bf v}(0) + \int_0^t {\bf a}(s) ds \, = \,2t\, {\bf i}+ 6t^2\, {\bf j} +{\bf v}(0)\, =\, 2t\, {\bf i} + 6t^2\, {\bf j} + 7\, {\bf i}\,.$$ Likewise, we integrate the velocity to get the position: $${\bf r}(t) \ = \ \int\, {\bf v}(t)\, dt \ = \ t^2\, {\bf i} + 2t^3\, {\bf j} + 7t\, {\bf i} + {\bf r}(0)\,.$$ Consequently, $${\bf r}(t) \ = \ t^2\, {\bf i} + 2t^3\, {\bf j} + 7t\, {\bf i} + (2\, {\bf i} + 9 {\bf k})$$ $$\qquad = \ (t^2 +7t +2)\, {\bf i} + 2t^3\, {\bf j} + 9\, {\bf k}\,,$$ or $$x(t) = t^2+7t+2; \qquad y(t) = 2t^3; \qquad z(t) = 9\,.$$

Chapter 10: Parametric Equations and Polar Coordinates

Chapter 12: Vectors and the Geometry of Space

Chapter 13: Vector Functions

Learning module LM 13.1/2: Vector valued functions

Learning module LM 13.3: Velocity, speed and arc length:

Learning module LM 13.4: Acceleration and curvature:

Chapter 14: Partial Derivatives

Chapter 15: Multiple Integrals

Position, velocity and acceleration