Main page
Chapter 10: Parametric Equations and Polar Coordinates
Learning module LM 10.1: Parametrized Curves:
Learning module LM 10.2: Calculus with Parametrized Curves:
Learning module LM 10.3: Polar Coordinates:
Learning module LM 10.4: Areas and Lengths of Polar Curves:
Learning module LM 10.5: Conic Sections:
Slicing a coneEllipses
Hyperbolas
Parabolas and directrices
Completing the square
Learning module LM 10.6: Conic Sections in Polar Coordinates:
Chapter 12: Vectors and the Geometry of Space
Chapter 13: Vector Functions
Chapter 14: Partial Derivatives
Chapter 15: Multiple Integrals
Ellipses
Ellipses can be elegantly described in four ways.
|
The simplest description of an ellipse is as a squashed or stretched circle. Start with the unit circle x2+y2=1, and stretch it by a factor of a in the x direction and b in the y direction to get:
| The standard formula for an ellipse in rectangular coordinates is x2a2+y2b2=1. |
|
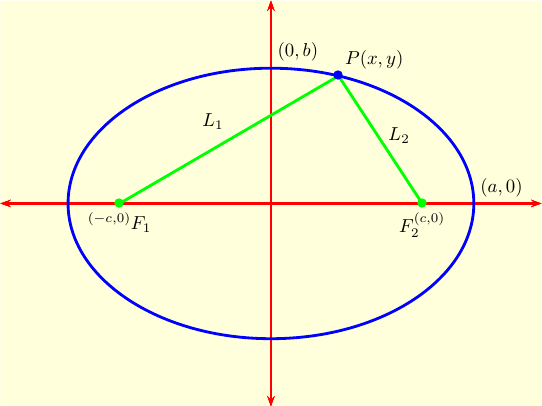
Now let c=√a2−b2. The points (±c,0) are called foci. These points are extremely important in astronomy, since planets follow elliptical orbits with the sun at a focus, not with the sun at the center. Let F1(−c,0) and F2(c,0) be the two foci, let P(x,y) be an arbitrary point on the ellipse. Let L1 be the distance from F1 to P, and let L2 be the distance from F2 to P, as in the figure on the right. |
|
| Amazing fact: The ellipse is the set of all points where L1+L2=2a. |
This fact gives elliptical rooms amazing acoustic properties. If you whisper at one focus of such a room, the sound waves from your voice will bounce off the walls and converge at the other focus -- that's why it is called a focus. The same goes for light reflecting off elliptical mirrors.
To understand the amazing fact, let's convert the equation L1+L2=2a to rectangular coordinates: L1+L2=2aL1=2a−L2√(x+c)2+y2=2a−√(x−c)2+y2(x+c)2+y2=4a2+(x−c)2+y2−4a√(x−c)2+y24a√(x−c)2+y2=4a2−4cxa√(x−c)2+y2=a2−cxa2(x−c)2+a2y2=a4+c2x2−2a2cxa2x2+a2c2−2a2cx+y2=a4+c2x2−2a2cx(a2−c2)x2+a2y2=a2(a2−c2)b2x2+a2y2=a2b2x2a2+y2b2=1, where we have used the fact that b2=a2−c2. That's a long and messy calculation for a simple and elegant result. You should be able to construct the equation of an ellipse given any two of a, b and c, since you can get the third from c2=a2−b2.
|
Example 1: Find the location of the foci of the ellipse
x225+y29=1.
|
Solution: We have a=5 and b=3, so c=√a2−b2=4. The foci are at (±4,0). |
|
Example 2: Find the equation of an ellipse
with foci at (±3,0) if b=4.
|
Solution: Since c=3 and b=4, a2=32+42=25, so a=5. This makes the equation x225+y216=1. |
|
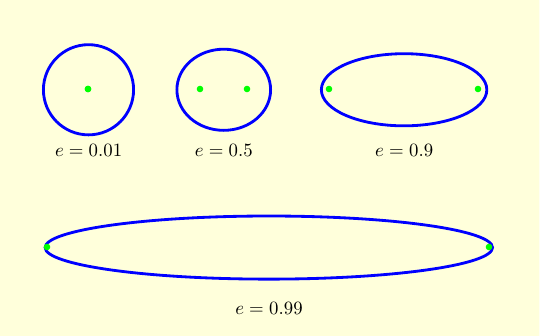
The ratio c/a is called the eccentricity of the ellipse, and is usually denoted e. Note that e<1. A circle can be viewed as an ellipse with eccentricity zero, and with both foci at the origin. |
|
| It is easy to plot an ellipse as a parametrized curve. Just take x=acos(t);y=bsin(t), with the parameter t running from 0 to 2π. |