Integrals over rectangles are
almost the same as integrals over intervals, except that now our
density $f(x,y)$ is the amount of stuff per unit area instead
of stuff per unit length. The simplest example of `stuff' is
volume, in which case $f(x,y)$ is height.
So suppose that $R = [a,b] \times [c,d]$ is a rectangle, where $x$ runs from $a$
to $b$ and $y$ runs from $c$ to $d$. Let's figure out the volume of the
solid between the $x$-$y$ plane and the surface $z=f(x,y)$, and over the
rectangle $R$.
Break the interval $[a,b]$ into $m$ pieces, each of size
$\Delta x = (b-a)/m$.
Break the interval $[c,d]$ into $n$ pieces, each of size
$\Delta y=(d-c)/n$.
Together, this breaks $R$ into $nm$ smaller rectangular boxes,
each of area $\Delta A =\Delta x \Delta y$. It breaks the solid
into little towers of width $\Delta x$, depth $\Delta y$, and
height $f(x,y)$.
Label the boxes with two indices $i,j$, where $i$ says what column
we're in and runs from 1 to $m$, while $j$ says what row we're in and
runs from 1 to $n$.
For each pair $i,j$, pick a sample point
$(x_{ij}^*, y_{ij}^*)$ somewhere in the $ij$-th box.
Approximate the volume of the tower over the $ij$-th box as
$f(x_{ij}^*,y_{ij}^*) \Delta A$.
Approximate the total volume as
$\displaystyle{\sum_{i=1}^m \sum_{j=1}^n f(x_{ij}^*, y_{ij}^*) \Delta A}$.
To get an exact answer, take a limit as $n \to \infty$ and
$m\to \infty$.
Investigate the interactive figure to the right. For each
function, the integral of
$f(x,y) dA$ is the volume of the region under the surface $z=f(x,y)$.
The volume of each tower is $f(x_{ij}^*, y_{ij}^*) \Delta x \Delta y$.
By varying the size of $\Delta x$ and $\Delta y$, and the choice of
representative points $(x_{ij}^*, y_{ij}^*)$, you can see how the sums
approximate the integral.
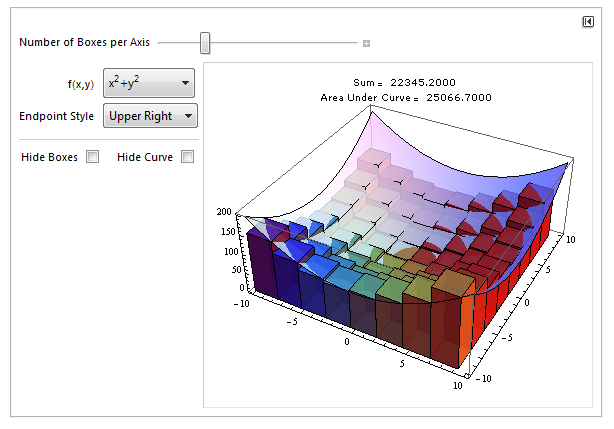As with functions of one variable, the limit of this sum is the
definition of a (double) integral.
If $f(x,y)$ is a continuous function of two variables and if $R = [a,b]
\times [c,d]$ is a rectangle, then the double integral of $f$ over $R$
is
$$\iint_R f(x,y) dA = \iint_R f(x,y) dx dy = \lim_{m \to
\infty}\lim_{n\to\infty} \sum_{i=1}^m \sum_{j=1}^n f(x_{ij}^*),
y_{ij}^*) \Delta A.$$
A convenient choice for the sample point $(x_{ij}^*,y_{ij}^*)$ is
given by the midpoint rule: $x_{ij}^* = a + (i-\frac12)\Delta x$,
$y_{ij}^* = c+(j-\frac12)\Delta y$.
In the following video, we develop the same definition with a slightly
different problem, namely computing the total population of the (conveniently
rectangular) state of Colorado.