Inverse Functions and Logarithms

Home

The Six Pillars of Calculus

The Pillars: A Road Map
A picture is worth 1000 words

Trigonometry Review

The basic trig functions
Basic trig identities
The unit circle
Addition of angles, double and half angle formulas
The law of sines and the law of cosines
Graphs of Trig Functions

Exponential Functions

Exponentials with positive integer exponents
Fractional and negative powers
The function $f(x)=a^x$ and its graph
Exponential growth and decay

Logarithms and Inverse functions

Inverse Functions
How to find a formula for an inverse function
Logarithms as Inverse Exponentials
Inverse Trig Functions

Intro to Limits

Overview
Definition
One-sided Limits
When limits don't exist
Infinite Limits
Summary

Limit Laws and Computations

Limit Laws
Intuitive idea of why these laws work
Two limit theorems
How to algebraically manipulate a 0/0?
Indeterminate forms involving fractions
Limits with Absolute Values
Limits involving indeterminate forms with square roots
Limits of Piece-wise Functions
The Squeeze Theorem

Continuity and the Intermediate Value Theorem

Definition of continuity
Continuity and piece-wise functions
Continuity properties
Types of discontinuities
The Intermediate Value Theorem
Summary of using continuity to evaluate limits

Limits at Infinity

Limits at infinity and horizontal asymptotes
Limits at infinity of rational functions
Which functions grow the fastest?
Vertical asymptotes (Redux)
Summary and selected graphs

Rates of Change

Average velocity
Instantaneous velocity
Computing an instantaneous rate of change of any function
The equation of a tangent line
The Derivative of a Function at a Point

The Derivative Function

The derivative function
Sketching the graph of $f'$
Differentiability
Notation and higher-order derivatives

Basic Differentiation Rules

The Power Rule and other basic rules
The derivative of $e^x$

Product and Quotient Rules

The Product Rule
The Quotient Rule

Derivatives of Trig Functions

Necessary Limits
Derivatives of Sine and Cosine
Derivatives of Tangent, Cotangent, Secant, and Cosecant
Summary

The Chain Rule

Two Forms of the Chain Rule
Version 1
Version 2
Why does it work?
A hybrid chain rule

Implicit Differentiation

Introduction
Examples
Derivatives of Inverse Trigs via Implicit Differentiation
A Summary

Derivatives of Logs

Formulas and Examples
Logarithmic Differentiation

Derivatives in Science

In Physics
In Economics
In Biology

Related Rates

Overview
How to tackle the problems
Example (ladder)
Example (shadow)

Linear Approximation and Differentials

Overview
Examples
An example with negative $dx$

Differentiation Review

How to take derivatives
Basic Building Blocks
Advanced Building Blocks
Product and Quotient Rules
The Chain Rule
Combining Rules
Implicit Differentiation
Logarithmic Differentiation
Conclusions and Tidbits

Absolute and Local Extrema

Definitions
The Extreme Value Theorem
Critical Numbers
Steps to Find Absolute Extrema

The Mean Value and other Theorems

Rolle's Theorems
The Mean Value Theorem
Finding $c$

$f$ vs. $f'$

Increasing/Decreasing Test and Critical Numbers
Process for finding intervals of increase/decrease
The First Derivative Test
Concavity
Concavity, Points of Inflection, and the Second Derivative Test
The Second Derivative Test
Visual Wrap-up

Indeterminate Forms and L'Hospital's Rule

What does $\frac{0}{0}$ equal?
Examples
Indeterminate Differences
Indeterminate Powers
Three Versions of L'Hospital's Rule
Proofs

Optimization

Strategies
Another Example

Newton's Method

The Idea of Newton's Method
An Example
Solving Transcendental Equations
When NM doesn't work

Anti-derivatives

Antiderivatives
Common antiderivatives
Initial value problems
Antiderivatives are not Integrals

The Area under a curve

The Area Problem and Examples
Riemann Sum Notation
Summary

Definite Integrals

Definition of the Integral
Properties of Definite Integrals
What is integration good for?
More Applications of Integrals

The Fundamental Theorem of Calculus

Three Different Concepts
The Fundamental Theorem of Calculus (Part 2)
The Fundamental Theorem of Calculus (Part 1)
More FTC 1

Logarithms as Inverse Exponentials

Throughout suppose that $a>1$. The function $y=\log_a(x)$ is the inverse of the function $y=a^x$. In other words,

$$\log_a(a^x) = x \qquad \hbox{and} \qquad a^{\log_a(x)}=x$$

whenever these make sense.

The value of $\log_b(a)$ is the power you raise $b$ to to get $a$.

Examples:

Since $10^3=1000$, $\log_{10}(1000)=3$.

Since $2^{-3}=1/8$, $\log_2(1/8)=-3$.

$10^{\log_{10}(17)}=17$ and $2^{\log_2(.038)}=.038$

Since $a^0=1$, no matter what $a$ is, $\log_a(1)=0$. In general $\log_a(x)$ will be positive for $x>1$ and negative for $x<1$.

The domain of $a^x$ is $(-\infty, \infty)$, so the range of $\log_a(x)$ is $(-\infty,\infty)$.

The range of $a^x$ is $(0,\infty)$, so the domain of $\log_a(x)$ is $(0,\infty)$. We cannot take the log of zero or the log of a negative number.

Laws of Logarithms

The laws of logs follow from the law of exponents. You should know these. Provided that $x>0$ and $y>0$ we have:

Logarithm Laws

$\log_{a}(xy) = \log_a(x) + \log_a(y)$

$\log_a(x/y) = \log_a(x) - \log_a(y)$

$\log_a(x^r) = r \log_a(x)$

$\log_b(a) = \frac{\log_x(a)}{\log_x(b)}$ for any valid log base $x$

The last item above is the "change of base" formula, which helps in approximating the value of logs using your calculator (which usually has only $\ln x$ and $\log x$ which are logs with base $e$ and $10$ respectively). So $\log_7(5)=\frac{\ln 5}{\ln 7}=\frac{\log 5}{\log 7}$, either of which you can compute using your calculator. These ratios are equal -- do not think that $\ln 5=\log 5$! The power you raise $e$ to to get 5 is not the same as the power you raise $10$ to to get 5.

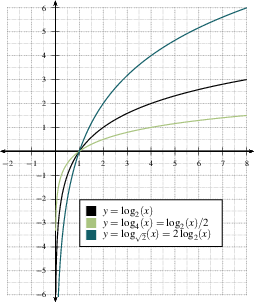
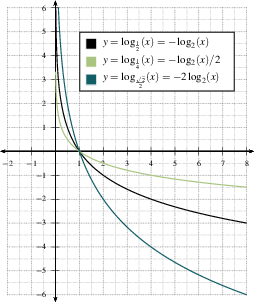
Graphs

Below are the graphs of the log functions when $a>1$ or $0 < a<1$.