Trig Substitution

Home

Examples

In this video, we work three examples, one with $x=a \tan(\theta)$, one with $x = a\sin(\theta)$, and one with $x = a \sec(\theta)$. Worked out solutions are written below the video.

Examples from the video.

DO: After watching the video, write down and work these examples on your own, slowly, thinking of the whys and hows of each step.

Example 1: $\int \bigl(4+x^2\bigr)^{-3/2}\, dx$
DO: Work through before looking ahead.

Solution 1: $\displaystyle\int \bigl(4+x^2\bigr)^{-3/2}\, dx \overset{\fbox{$ \,\, x\,=\,2\tan\theta\\dx\,=\,2\sec^2\theta\,d\theta$}}{=}\int \bigl(4 + 4\tan^2\theta\bigr)^{-3/2} 2 \sec^2\theta \,d\theta=\int \bigl(4 \sec^2\theta\bigr)^{-3/2} 2 \sec^2(\theta) \,d\theta$

$\displaystyle =4^{-3/2}\cdot 2\int\sec^{-3}\theta\sec^2\theta\,d\theta=\frac{2}{8}\int\sec^{-1}\,d\theta=\frac{1}{4}\int \frac{d\theta}{\sec\theta} = \frac{1}{4}\int \cos\theta\, d\theta = \frac{1}{4}\sin\theta+C.$

Consider our answer above. In order to convert back into terms of $x$, we must figure out what $\sin(\theta)$ is in terms of $x$. By rewriting our original substitution we see that $\tfrac x 2=\tan\theta$. Use this to draw a right triangle, with opposite side $x$ and adjacent side $a=2$. The hypotenuse is then $\sqrt{a^2+x^2}=\sqrt{4+x^2}$. We need to find $\sin\theta$ in terms of $x$, and we see from the triangle that $\sin\theta=\frac{x}{\sqrt{x^2+4}}$.

So $\displaystyle\int\left(4+x^2\right)^{-3/2}\,dx=\frac{1}{4}\sin\theta+C=\frac{x}{4\sqrt{x^2+4}}+C$.

---------------------------------------------------------------------------
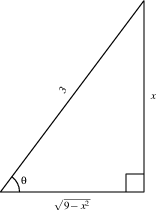
Example 2: $\int \sqrt{9-x^2}\, dx$
DO: Work through before looking ahead.

Solution 2: $\displaystyle\int \sqrt{9-x^2}\, dx\overset{ \fbox{$ \,\,x\,=\,3 \sin\theta\\dx\,=\,3\cos\theta\,d\theta$} }{=} \int \left(\sqrt{9-9\sin^2\theta}\right)3\cos\theta\,d\theta=3\cdot 3\int\sqrt{\cos^2\theta}\cos\theta \,d\theta=9\int\cos^2\theta \,d\theta$

$\displaystyle\quad =9\int\frac{1+\cos(2\theta)}{2}\,d\theta=\frac{9}{2}\int(1+\cos(2\theta))\,d\theta =\frac{9}{2} \left(\theta+\frac{\sin(2\theta)}{2}\right)+C=\frac{9}{2}\bigl(\theta+\sin\theta\cos\theta\bigr)+C $$

Here we have used the methods of the last learning module to evaluate the trig integral, including the handy trig identities for $\cos^2\theta$ and $\sin(2\theta)$. (You need to know these by heart). We look at the terms in our final answer above. We use the triangle to convert $\sin\theta\cos\theta$ back into terms of $x$. Finally, we must write $\theta$ in terms of $x$. We use our original substitution: $\frac{x}{3}=\sin x$ gives us $\sin^{-1}(\tfrac x 3)=\theta$.

So we have $\displaystyle\int \sqrt{9-x^2}\, dx=\frac{9}{2}\left( \sin^{-1} \left (\frac{x}{3} \right ) + \frac{x}{3}\frac{\sqrt{9-x^2}}{3}\right)+ C=\frac{9}{2} \sin^{-1} \left (\frac{x}{3} \right ) + \frac{x \,\sqrt{9-x^2}}{2} + C$

---------------------------------------------------------------------------
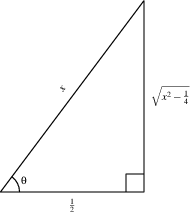
Example 3: $\displaystyle\int \frac{dx}{\sqrt{4x^2-1}}$
DO: Work through before looking ahead.

Solution 3: $\displaystyle\int \frac{dx}{\sqrt{4x^2-1}} \overset{ \fbox{$ 2x\,=\, \sec\theta\\2dx\,=\,\sec\theta\tan\theta\,d\theta$} }{=} \int \frac{\frac{1}{2}\sec\theta\tan\theta\,d\theta}{\sqrt{\sec^2\theta-1}}
\overset{ \fbox{$ \sec^2\theta-1\,=\,\tan^2\theta$} }{=} \frac{1}{2}\int \frac{\sec\theta\tan\theta\,d\theta}{\sqrt{\tan^2\theta}}$

$\displaystyle= \frac{1}{2}\int \frac{\sec\theta\tan\theta\,d\theta}{\tan\theta}=\frac{1}{2}\int\sec\theta\,d\theta=\frac{1}{2} \ln\bigl\lvert\sec\theta+\tan\theta\bigr\rvert +C. $

We now convert to terms of $x$: $\sec\theta=2x$, so $\cos\theta=\frac{1}{2x}$. The triangle could therefore have adjacent side of 1, and hypotenuse of $2x$, and the opposite side $\sqrt{4x^2-1}$. We can also have adjacent of $\frac{1}{2}$ and hypotenuse of $x$. (Why?) This gives the opposite side the value of $\sqrt{x^2-\frac{1}{4}}$, as in the diagram. Either way is fine. Looking at our values above, we only need deal with $\tan \theta$, since we know $\sec\theta=2x$. From the triangle, $\tan\theta=\frac{\sqrt{x^2-1/4}}{1/2}$. If we had used the other triangle, we would get $\tan\theta=\frac{\sqrt{4x^2-1}}{1}$ -- are these the same values?

So $\displaystyle\int \frac{dx}{\sqrt{4x^2-1}} =\frac{1}{2} \ln \bigl\lvert \,2x + \sqrt{4x^2-1}\,\bigr\rvert + C.$

The Fundamental Theorem of Calculus

The Indefinite Integral and the Net Change

Substitution

Area Between Curves

Volumes

Integration by Parts

Integrals of Trig Functions

Trig Substitutions

Partial Fractions

Strategies of Integration

Improper Integrals

Differential Equations

Models of Growth

Infinite Sequences

Infinite Series

Integral Test

Comparison Tests

Convergence of Series with Negative Terms

The Ratio and Root Tests

Strategies for testing Series

Power Series

Representing Functions as Power Series

Taylor and Maclaurin Series

Applications of Taylor Polynomials

Partial Derivatives

Multiple Integrals

Iterated Integrals over Rectangles

Double Integrals over General Regions

Examples

Examples from the video.